Typical Floor Framing Element Check:

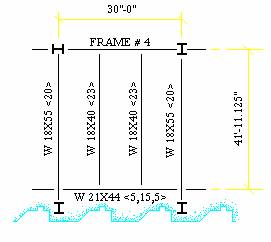
The typical floor frame consists of W18x40 beams with 23 shear studs and a 10-foot tributary width as well as W21x44 girders with 20 shear studs. This framing plan was taken between column lines 7, 8 and D, B, which consists primarily of two equally spaced beams and two girders. The composite frame consists of a lightweight concrete slab, with mesh reinforcing, a steel deck, and shear studs. The case study, of the load vs. member capacity, calculations are located in the appendix using LRFD. This method was also implemented by the structural engineer for this project. The beam and girder that were check meet the requirements of the load, however an accurate calculation of the required number of shear studs could not be accurately determined from the information available from the project drawings and specifications. The flowing data and assumptions were used in the calculations performed.

Data from Drawings and Specifications:

● Concrete:

- F’c= 3000 psi

- 3.25” Light weight concrete slab

● Steel Decking:

- 3” 20 gage steel deck

- Thickness = 0.0474”

- Integrally embossed or raised pattern ribs

- Interlocking side laps

- Thickness = 0.0474”

● Shear Studs:

- 0.75” x 5.25” head studs

- Welded to the top of beam or girder flange.

- Typically placed in a single row centered over beam web.

Assumptions:

● The system was assumed to be fully composite.

● 25-psf-partition load

● 2.7-psf deck weight

● 5 psf of reinforcing steel in concrete slab.

● Used 6.25” to calculate weight of lightweight concrete slab.

● Deck rib width = 2.5”.

● Final stud height after welding = 5.0”.

Beam and Girder Calculations:

I used the fully composite beam calculation method. I found the loads that would be placed on these members from the load requirement found in ASCE 7-98. I then found the capacities of the concrete and steel beams, choosing the lower value, the steel, for the shear transfer amount. Next I proceed to find the “a” value that would give the equivalent stress, and found the moment capacity with a safety factor of 0.85.

Results:

Composite Beam

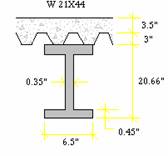
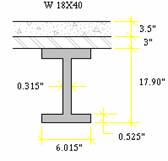The results for the following calculations can be found in the Appendix. The moment applied to the beams 599.88 ft kips and the capacity of the composite slab assuming that it was the beam was fully composite totaled 594.9 ft kips, which is slightly below the amount that is needed for the loads. This difference might be due to my calculation of the slab weight. Revisions were made to take into account that voids that be caused by the steel deck and the moment produced by the loads will be significantly less, approximately 560 ft kips. However it is unknown at the time that exact steel deck that was used in construction.

The moment applied to the girders was due to the live and dead loads were 662.6 ft kips. At the time there is not enough information about the exterior wall loads, as a result they were estimated to be 20 psf. The capacity of the girder was sufficient to carry this load equaling 698.2 ft kips. Thus the W 21x44 chosen for the design was appropriate.

Composite Girder

Error in Shear Stud Calculations:

This error is not the result of incorrect assumptions for the deck rib width, and stud height after welding. The Q_n is equal to 18.25 kips for lightweight concrete with a 3 inch concrete. When the value for Q_n was calculated by dividing the moment by 23 studs the Q_n = 25.7 which was greater than the value available. Thus, even if the assumed values produced a reduction factor that was equal to one, the nominal stud strength would have been insufficient. The error was most likely due to the assumption that the beams are fully composite. A lower transfer moment would decrease the amount of shear studs needed. The design, with the lower concrete weight, would have allowed room for a lower moment capacity. However, I was unable to determine the extent at which the member was partially composite before the completion of this project.